Gas-Liquid Separators Sizing Parameter

Download PDF Version

In the December 2014 tip of the month (TOTM) [1], we discussed troubleshooting of gas-liquid separators for removal of liquids from the gas stream leaving the separator. There are two methods for sizing gas-liquid separators: 1. Droplet settling theory method, 2. Souders-Brown approach. Historically the Souders-Brown equation has been employed as it can provide reasonable results and is easy to use, but has shortcomings in terms of quantifying separator performance.  References [2-4] provide the details on the droplet settling theory methods which can be used to more accurately quantify separator performance.  The Souders-Brown method is limited in that it is based on the average droplet size, but cannot quantify the amount of liquid droplets exiting the gas gravity section.

In this TOTM, we will focus on the application of Souders-Brown approach in gas-liquid separators and present diagram, simple correlations and tables to estimate the Souders-Brown equation constant, K_S (the so called sizing parameter). We will consider both vertical and horizontal gas-liquid separators. Knowing the actual gas flow rate through the vessel, one can use K_S parameter to determine the maximum allowable gas velocity through the vessel and determine the required separator diameter. One can also use the appropriate value of K_S to size the mist extractor in the vessel. The performance of a gas-liquid separator is highly dependent on the value of K_S; therefore, the choice of appropriate  K_S –values is important.

Gas Gravity Separation Section

The gas gravity separation section of a separator has two main functions:

1. Reduction of entrained liquid load not removed by the inlet device
2. Improvement / straightening of the gas velocity profile.

Most mist extractors have limitations on the amount of entrained liquid droplets that can be efficiently removed from the gas, thus the importance of the gas gravity section to remove the liquids to an acceptable level upstream of the mist extractor.  This is particularly important for separators handling higher liquid loads. For scrubber applications with low liquid loadings,  the K_S –values will be primarily dependent on the mist extractor type, and the gas gravity separation section becomes less important. For the higher liquid load applications, i.e. conventional separators, there are two approaches for sizing the gravity separation section to remove liquid droplets from the gas:

1. The Souders-Brown approach (K_s Method)
2. Droplet settling theory

The Souders-Brown Approach

If we consider a spherical liquid droplet with a diameter of D_P in the gas phase two forces as shown in Figure 1 act on it. The drag force, F_D, is exerted by flow of gas and gravity force, F_G, is exerted by the weight of droplet. The drag force acts to entrain the liquid droplet while the gravity force acts to pull it down and separating it from the gas phase.

Figure 1. Schematic of the forces acting on a liquid droplet in the gas phase [5]

       (1)

As presented in the Appendix, substitution of expressions for the drag and gravity forces in Equation 1, the maximum allowable gas velocity, V_Gmax, which prevents entrainment of liquid is obtained.

Equation 2 is called Souders-Brown [6] equation and K_S is referred to as the design or sizing parameter. The terms ρ_G and ρ_L are the gas phase and liquid phase densities, respectively.

Once the maximum gas velocity, V_Gmax, through the vessel is determined by Equation 2, one can calculate the minimum vessel diameter, D_min by Equation 3.

Where:

F_G        = Fraction of cross section area available for gas flow (F_G = 1 for vertical separators and is a function of liquid height for horizontal separators)

q_a         = Gas flow rate at the actual flowing condition

The Design Parameter, K_S

The design parameter, K_S, in the Souders-Brown equation is an empirical parameter and is a key factor for the sizing the gas-liquid separators vessel diameter as well as for determination of the mist extractor diameter. Its value depends on several factors including:

• Pressure
• Fluid properties (note temperature has a large impact on fluid properties)
• Separator geometry
  • Vessel length and the liquid level (for horizontal separators)
• Steadiness of flow
• Inlet device design and performance
• Relative amounts of gas and liquid
• Most importantly – mist extractor type and design (e.g. mesh pad, vane pack, multi–cyclone)

There are several sources that one can look up the K_S –values for different applications.  In the following sections, we will discuss three sources.

A. API 12 J

The API 12J [7] recommends ranges of K_S –values for vertical and horizontal gas-liquid separators. These values are presented in Table 1. The equivalent of API 12J for the North Sea region is NORSOK P-100.

Table 1. API 12 J recommended range of K_S –values for vertical and horizontal separators [7]

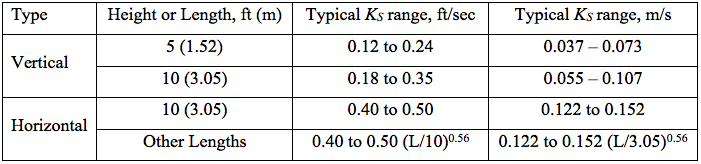
Per API 12J, “the maximum allowable superficial velocity, calculated form the above factors, is for separators normally having a wire mesh mist extractor. This rate should allow all liquid droplets larger than 10 microns to settle out of the gas. The maximum allowable superficial velocity or other design criteria should be considered for other type mist extractor. Mist extractor manufacturer’s recommended minimum distances upstream and downstream of the wire mesh between gas inlet and outlet nozzles should be provided for full utilization of the mist extractor. These values assume separators are equipped with standard mesh pad mist extractors” [7].

B. Campbell Book

The K_s method, Equation 2, is an empirical approach to estimate the maximum allowable gas velocity to achieve a desired droplet separation. For vertical separators with no mist extractor devices, Chap 11, Vol 2 of the Gas Conditioning and Processing book presents K_S as a function of pressure and liquid droplet size [5]. This dependency of K_S on pressure and droplet size is presented in Figure 2 [5]. Note for each droplet size a range of K_S –values are given for a specified pressure.

For horizontal separators, the sizing depends on (in addition to the droplet size, density of gas and liquid phases, and gas velocity) separator effective length, L_e, and the depth available for gas flow, h_G, (i.e. liquid level) in the separators.

Figure 2. KS as a function of pressure and liquid droplet size for vertical separators with no mist extractor devices [5]

Sizing of the horizontal separators are more complicated. Referring to Figure 3, the effective L_e may be defined in terms of separator actual length and diameter like L_e=L-D. Therefore, the Souders-Brown parameter for horizontal separators, K_SH, can be estimated in by Equation 4 in terms of K_SV (read from Figure 2) for vertical separator [3].

If the calculated value of K_SH by Equation 4 is greater than the maximum value of 0.7 ft/sec (0.21 m/s), it should be set equal to this maximum value.

Figure 3. Schematic of a horizontal gas-liquid separator [5]

Where:

D         = Diameter

F_L        = Fraction of cross section area occupied by liquid (function of liquid height in horizontal separator)

q_L         = Liquid actual volume flow rate

t           = Residence time per API 12J [7]

If the calculated L/D is outside of its recommended range (normally 3 < L/D < 6), the liquid height in the vessel is changed and the calculations are repeated. For detail of calculations procedure refer to chapter 11 of reference [5].

C. K_S Correlations

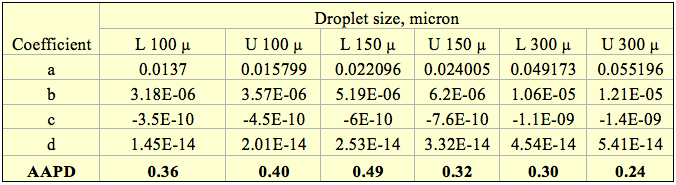
The curves for different droplet sizes shown in Figure 2 are fitted to a 3^rd order polynomial (for droplet sizes of 100, 150, and 300 microns). The correlation is in the form of Equation 6 and its regressed coefficients a, b, c, and d are presented in Tables 2A and 2B for field (FPS) and System International (SI) units, respectively.

In Table 2, each droplet size in micron (µ) is preceded by letter L or U representing the lower and upper curve, respectively. The pressure is in psi and K_S is in ft/sec for FPS (kPa and m/s in SI).

The last row of Table 2 provides the average absolute percent deviation (AAPD) of the predicted K_S by the proposed correlation from the corresponding values of Figure 2 values.

Table 2A (FPS). Regressed coefficients for Equation 6 (P in psi and K_S in ft/sec)
Droplet Size: 100 – 300 microns

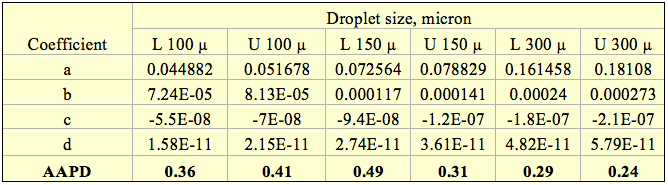
Table 2B (SI). Regressed coefficients for Equation 6 (P in kPa and K_S in m/s in SI).
Droplet Size: 100 – 300 microns

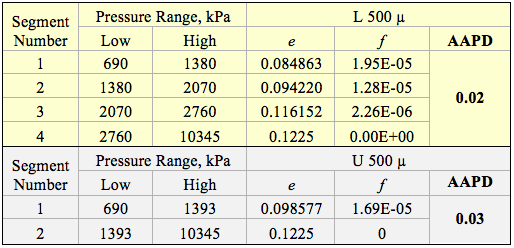
The two curves for 500 micron droplet size in Figure 2 were divided into 4 and 2 segments based on pressure range for the lower and upper curves, respectively. Each segment was fitted to a linear equation in the form of Equation 7 and its regressed coefficients e and f are presented in Tables 3A and 3B for FPS and SI units, respectively.

Table 3A (FPS). Regressed coefficients for Equation 7 (P in psi and K_S in ft/sec)
Droplet Size: 500 microns

Table 3B (SI). Regressed coefficients for Equation 7 (P in kPa and K_S in m/s in SI).
Droplet Size: 500 microns

D. Mist Extractors

The mist extractor is the final gas cleaning device in a conventional separator.  The selection, and design to a large degree, determine the amount of liquid carryover remaining in the gas phase.  The most common types include wire mesh pads (“mesh pads”), vane-type (vane “packs”) and axial flow demisting cyclones.  Figure 4 shows the location and function of a typical mist extractor in a vertical separator.

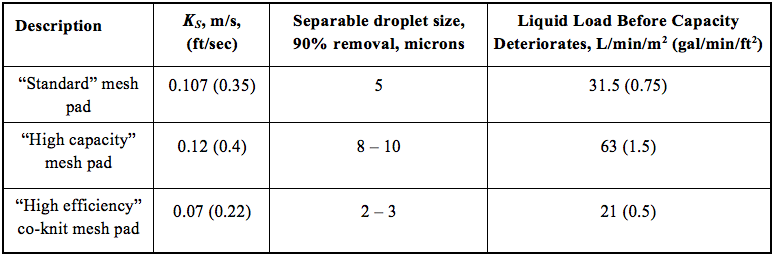
Mist extractor capacity is defined by the gas velocity at which re-entrainment of the liquid collected in the device becomes appreciable.  This is typically characterized by a K_S –value, as shown in Equation 2.  Mesh pads are the most common type of mist extractors used in vertical separator applications.  The primary separation mechanism is liquid impingement onto the wires, followed by coalescence into droplets large enough to disengage from the mesh pad.  References [1-5] provide mesh pad examples.  Table 4 provides a summary of mesh pad characteristics and performance parameters.

Figure 4. Typical mist extractor in a vertical separator [5]

Table 4. Mesh pads K_S and performance parameters [3, 5, 8]

Notes:

• Flow direction is vertical (upflow).
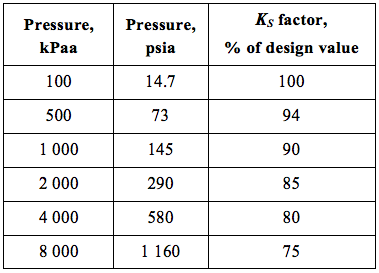
• Assume mesh pad K_S – value decline with pressure as shown in Table 5. Table 5 was originally developed for mesh pads, but is used as an approximation for other mist extractor types. [9].
• If liquid loads reaching the mesh pad exceed the values given in Table 4, assume capacity (K_S) decreases by 10% per 42 L/min/m² (1 gal/min/ft²). [2-4].
• These parameters are approximate.

Table 5. Mesh pad K_S deration factors as a function of pressure [5]

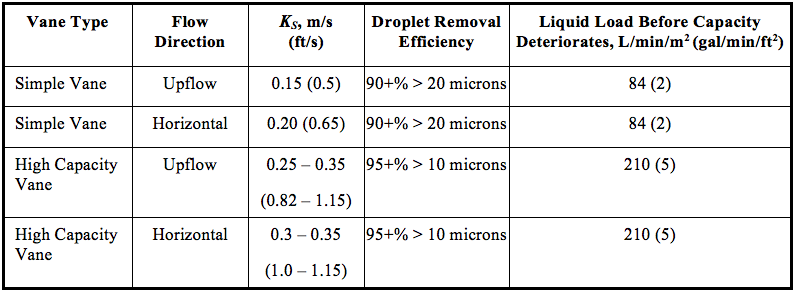
Vane packs, like mesh pads, capture droplets primarily by inertial impaction.  The vane bend angles force the gas to change direction while the higher density liquid droplets tend to travel in a straight-line path, and impact the surface of the vane where they are collected and removed from the gas flow. Table 6 provides vane pack performance characteristics [3, 5, 8].

In the case of demisting cyclones, the vendor should be consulted in regards to performance for the current operations of interest.

Table 6. Typical vane-pack characteristics [3, 5, 8]

Notes:

1. Assume vane-pack K_S – value decline with pressure as shown in Table 5.
2. If liquid loads reaching the vane pack exceed the values given in Table 2, assume capacity K_S decreases by 10% per 42 L/min/m² (1 gal/min/ft²). [2-4].
3. These parameters are approximate only. The vane-pack manufacturer should be contacted for specific information.

Conclusions: 

We focused on the application of Souders-Brown Approach (SBA) in gas-liquid separators and presented diagram, simple correlations and tables to estimate the SBA design parameter, K_S.

• The SBA can provide reasonable results and is easy to use.
• The SBA is limited in that it is based on the average droplet size, but cannot quantify the amount of liquid droplets exiting the gas gravity section and mist extractor section.
• In a future TOTM we will discuss the droplet settling theory methods which can be used to more accurately quantify separator performance.
• Sizing of three-phase gas-liquid hydrocarbon-liquid water separators are more complicated and will be discussed in another TOTM.

To learn more about similar cases and how to minimize operational problems, we suggest attending our PF49 (Troubleshooting Oil and Gas Facilities), PF42 (Separation Equipment Selection and Sizing), G4 (Gas Conditioning and Processing), G5 (Gas Conditioning and Processing – Special), and PF4 (Oil Production and Processing Facilities), courses.

PetroSkills offers consulting expertise on this subject and many others. For more information about these services, visit our website at http://petroskills.com/consulting, or email us at consulting@PetroSkills.com.

Dr. Mahmood Moshfeghian

References:

1. Snow–McGregor, K., http://www.jmcampbell.com/tip-of-the-month/2014/12/troubleshooting-gas-liquid-separators-removal-of-liquids-from-the-gas/
2. Bothamley, M., “Gas-Liquid Separators – Quantifying Separation Performance Part 1,” SPE Oil and Gas Facilities, pp. 22 – 29, Aug. 2013.
3. Bothamley, M., “Gas-Liquid Separators – Quantifying Separation Performance Part 2,” SPE Oil and Gas Facilities, pp. 35 – 47, Oct. 2013.
4. Bothamley, M., “Gas-Liquid Separators – Quantifying Separation Performance Part 3,” SPE Oil and Gas Facilities, pp. 34 – 47, Dec. 2013.
5. Campbell, J.M., Gas Conditioning and Processing, Volume 2: The Equipment Modules, 9^th Edition, 2^nd Printing, Editors Hubbard, R. and Snow–McGregor, K., Campbell Petroleum Series, Norman, Oklahoma, 2014.
6. Souders, M. and Brown, G. G., “Design of Fractionating Columns-Entrainment and Capacity,” Industrial and Engineering Chemistry, Volume 26, Issue 1, p 98-103, 1934.
7. American Petroleum Institute, 12J, Specification for Oil and Gas Separators, 8th Edition, October, 2008.
8. PF-49, Troubleshooting Oil and Gas Processing Facilities, Bothamley, M., 2014, © PetroSkills, LLC. All Rights reserved.
9. Fabian, P., Cusack, R., Hennessey, P., Neuman, M., “Demystifying the Selection of Mist Eliminators, Part 1: The Basics,” Chem Eng 11 (11), pp. 148 – 156, 1993.

Appendix

Derivation of the Souders-Brown and Stokes’ Law Equations

If we consider a spherical liquid droplet with a diameter of, D_P, in the gas phase two forces as shown in Figure 1 act on it. The drag force, F_D, is exerted by flow of gas and gravity force, F_G, is exerted by weight of droplet. The drag force acts to entrain the liquid droplet while the gravity force acts to pull it down.

Figure 1. Schematic of the forces acting on a liquid droplet in the gas phase [5]

At equilibrium, these two forces are equal.

The drag force is expressed as:

The droplet projected area, A_P, is defined by:

The gravity force, F_G, is defined

The volume of spherical droplet, V_D, is calculated by

Substitution of Equations 3 and 4 into Equation 1 and solving for the gas maximum velocity,

For practical applications, the first term on the right hand side is replaced by K_S

Therefore, the maximum gas velocity which prevents entrainment of liquid is obtained.

Equation 6 is called Souder-Brown equation and K_S is referred to as the design parameter.

Where:

A_P        = Project area of droplet

C_D        = Drag coefficient

g          = Acceleration of gravity

g_C        = Conversion factor

V          = Gas velocity

V_P        = Volume of droplet

ρ_G        = Gas density

ρ_L         = Liquid density

Once the maximum, V_Gmax, gas velocity through the vessel is determined by Equation 6, one can calculate the required minimum cross sectional area of vessel for gas flow by the following equation.

Solving for the minimum vessel diameter, D_min.

Where:

F_G        = Fraction of cross sectional area available for gas flow (F_G = 1 for vertical separators and it is a function of liquid height for horizontal separators)

q_a         = Gas flow rate at the actual flowing condition

The drag coefficient, C_D, is a function of Reynolds number, Re=(D_PVρ_G)/µ_G. For Stokes’ law Re <≈2

Substitution of C_D from Equation 9 into Equation 4 gives liquid droplet terminal velocity, V_T, in the gas phase based on the Stokes’ law.

Similarly, the terminal velocity for the other flow regimes like Intermediate and Newton can be derived based on their corresponding expressions for the drag coefficients [3].

Download PDF Version