In this Tip of the Month, we look at how to deal with some of the challenges of managing process safety. This TOTM is an excerpt of a paper presented by JMC Instructor/Consultant, Clyde Young at the 2008 Mary K. O’Connor Process Safety Symposium. This TOTM continues where the February 2009, TOTM left off.
When there are newspaper accounts of process incidents that have occurred, there is usually a statement along the lines of, “It just happened with no warning.” There are warning signs for every incident. Latent failures exist in all processes and eventually lead to active failures when circumstances align. Personnel must be taught how to see and react to these warning signs.
Throughout the lifecycle of a process, many tasks are performed. Even when a process is running in “normal” mode, operators perform routine tasks and maintenance to keep the process at “normal”. Now and then, the process is shut down for maintenance and then started again. Every time a task is performed there is the possibility that a latent condition may expose itself and lead to an active failure. Many organizations have implemented a requirement that all job tasks be analyzed through a process known as Job Task Analysis (JTA), Job Safety Analysis (JSA), or Job Hazard Analysis (JHA). There are many titles and acronyms for this process, but all have one common theme. Analyze the task to be performed, identify hazards and mitigate those hazards. Sadly, these analyses become routine and the documentation associated with them becomes nothing more than a checklist that needs to be filled out and turned in. This is sometimes known as “pencil whipping” the form.
Performing a job hazard analysis is not difficult, but does need to be a formalized process that controls or eliminates the hazards identified. This is the third simple thing we can do to improve our process safety management systems.
Review the checklist below:
- PROCEDURES
- What are the procedures for the task?
- What is unclear about the procedures?
- What order will we use these procedures?
- What permits are needed for hazard controls?
- EQUIPMENT AND TOOLS
- What are the right tools for the job?
- What is the correct way to use them?
- What is the condition of each tool?
- POSITIONS OF PEOPLE
- What could we be struck by?
- What could we strike ourselves against?
- What can we get caught in/on/between?
- What are potential trip/fall hazards?
- What are potential hand/finger pinch points?
- What extreme temperatures will we be in/around?
- What are the risks of inhaling, absorbing, swallowing hazardous substances?
- What are the noise levels?
- What electrical current/energized system could we come in contact with?
- What would be a cause for overexerting ourselves?
- PERSONAL PROTECTIVE EQUIPMENT (PPE)
- What is the proper PPE?
Hard hat, glasses/goggles, ear plugs, gloves, steel toe boots, respiratory system, fire retardant clothing
- CHANGING THE COURSE OF WORK
- What would cause us to have to stop or rearrange the job?
- What would cause us to change our tools or equipment?
- What would cause us to have to change our position?
- What would cause us to have to change our PPE?
YOU HAVE THE RIGHT AND
THE OBLIGATION TO
STOP UNSAFE ACTS
The above checklist is being used by a major oil and gas production company and has become a key element of how they do things. In other words, it is part of their culture. Contractors working for this company have begun using the checklist to analyze the tasks they perform.
The procedure for using the checklist is simple. All personnel assigned to perform a task will gather for a meeting. Each person has a copy of this checklist and one person will be assigned to document the findings of the meeting. A leader is assigned and the leader begins asking the questions, in the order written. The group answers each question and all the answers are documented. This is vital because if the process is not documented, it did not happen. Each group member follows along with the checklist and it is their responsibility to insure that the leader does not skip a question or that any member does not fail to answer a question.
Consider the first question, “What are the procedures for the task?” Answering this question will require that the appropriate procedures are gathered. The second question, “What is unclear about the procedures?” will insure that all personnel have reviewed the procedures. If there is no written procedure, then one must be created.
As the checklist is reviewed and each question answered and documented, a thorough review of the job will be conducted and any hazards or issues identified will be mitigated or addressed. In the end, all personnel will become more competent at identifying and mitigating hazards. Latent failures may be exposed and the job can proceed safely.
Some may say, “Wait a minute here. Conducting JHAs is usually considered a personnel safety issue and we know that having a good personnel safety record does not indicate effective process safety.” This is true, but one of the elements of risk based process safety is safe work practices. On many occasions, process incidents begin with routine job tasks that are not performed correctly. Using the JHA checklist according to a formalized procedure yields several benefits. Personnel performing the jobs have the necessary procedures for performing the task. The procedures are reviewed to insure accuracy. Procedures are identified for development. Training issues are identified for personnel who do not understand the procedures or task. Hazards that are not readily apparent are identified and mitigated before the job. Latent failures are identified and addressed. Deviations from “normal” can be predicted and addressed early in a project or task. Even if an organization has implemented a global JHA process, local management can use this JHA checklist to enhance the organization’s process.
Performing a JHA with this checklist may be a bit time consuming at first. As personnel become more familiar with and practice the process, the time required will be reduced. The analysis of each job will take as long as necessary to do a thorough review. Even though production pressures are always part of every job, whatever time is required to do an effective analysis will be worth it.
The three simple things presented in this paper are meant to be implemented at the process/plant level, not at the global level of an organization. Implementing them at the process and plant level is much like a pilot project and the process of implementation can be more easily fine tuned. Effective process safety management system implementation and maintenance can be difficult and time consuming. These simple things can be modified as personnel become more competent and thus make management of process safety more efficient and effective.
The Center for Chemical Process Safety (CCPS) book, “Guidelines for Risk Based Process Safety”, concludes with the following [1]:
“Standing still, congratulating ourselves on the successes of the past 20 years, and celebrating accidents that did not occur because of all of our hard work, will not prevent the next accident. Improvement will always be necessary. We must choose between moving forward, standing still, or slipping backward. We need not debate which direction to choose, only embrace the opportunity for each company to make a risk informed decision regarding which forward path leads more directly to the ultimate goal of a safe, effective, and economically competitive operation.”
Too often it is heard that the reason something is done a certain way is because it’s always been done that way. That does not mean the way things are done is correct or efficient. These three simple things may seem onerous at first, but they do not have to be permanent changes. They only need to be implemented long enough to insure personnel are competent and efficient at process safety. This is especially important when it is considered that over the next 10 years it is estimated that the oil and gas industry will be required to replace everyone who was hired in the early 1980’s.
The next generation of workers in our industry needs to be given every opportunity to become competent at process safety.
If you would like a copy of the paper that was presented, please contact John M. Campbell & Co. and request a copy.
To learn more about managing process safety systems, we suggest attending our PetroSkills HSE course, HS 45- Risk Based Process Safety Management or schedule a session of our two day Process Safety Case Study for Operations and Maintenance – OT 21, which can be found in our catalog. To enhance process safety engineering skills we suggest any of the JMC foundation courses or of our newly developed, PS 4 – Process Safety Engineering course.
By: Clyde Young
Instructor/Consultant
Reference:
- Center for Chemical Process Safety (CCPS) , “Guidelines for Risk Based Process Safety”, http://www.wiley.com/WileyCDA/WileyTitle/productCd-0470165693.html

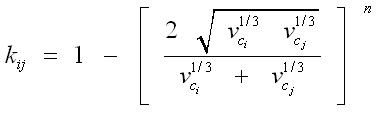
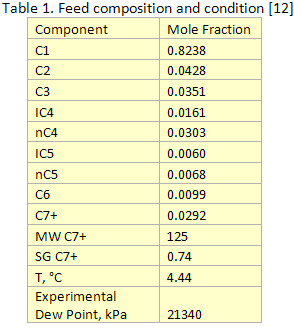

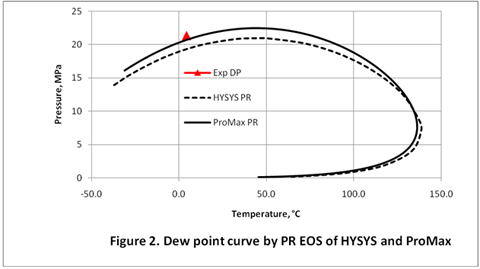
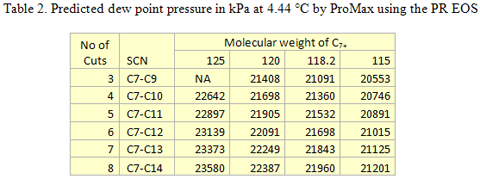
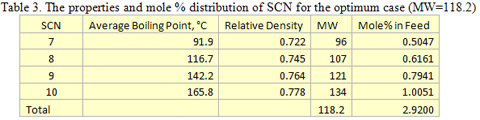
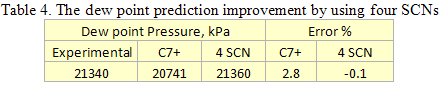
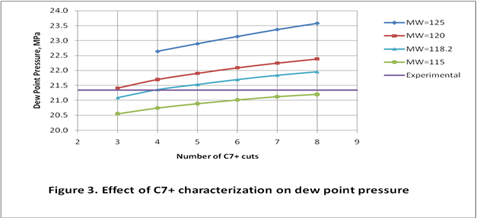
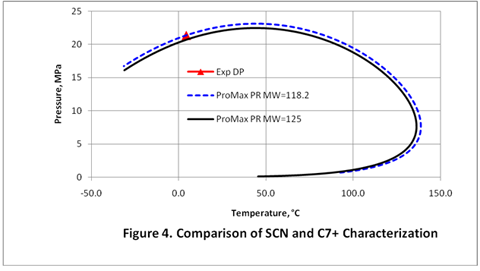




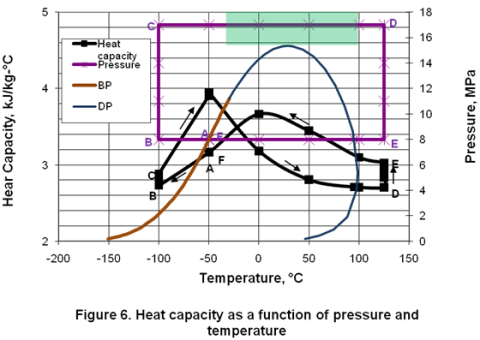
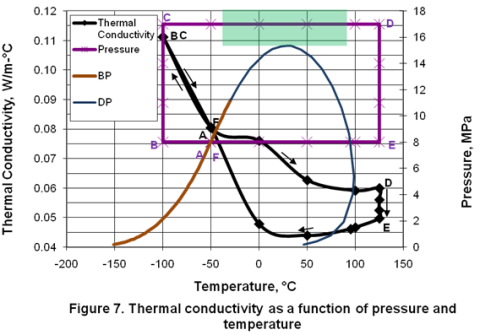
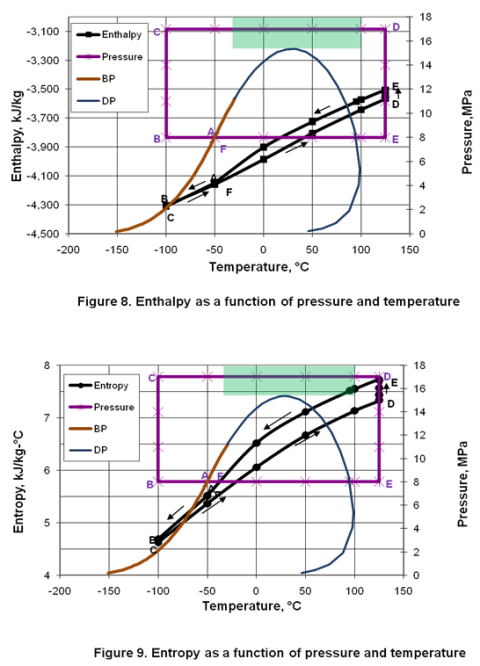
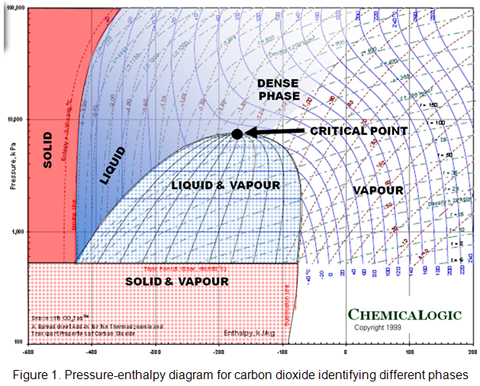


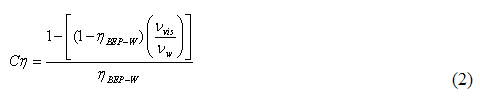

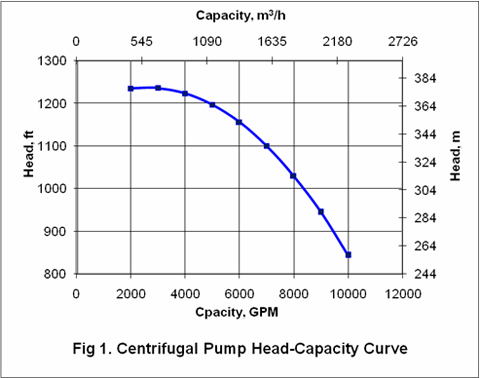
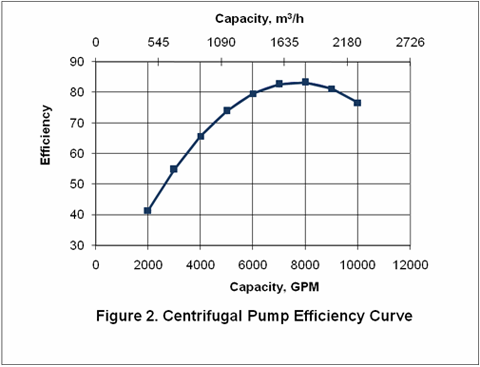

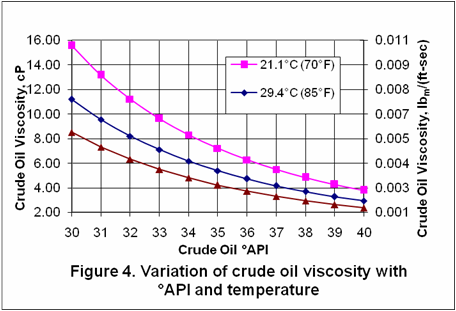
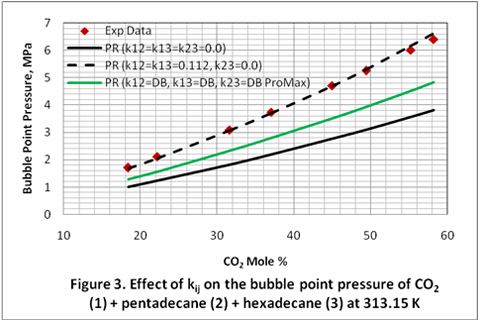

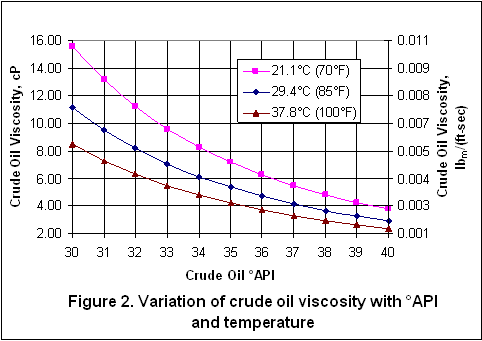
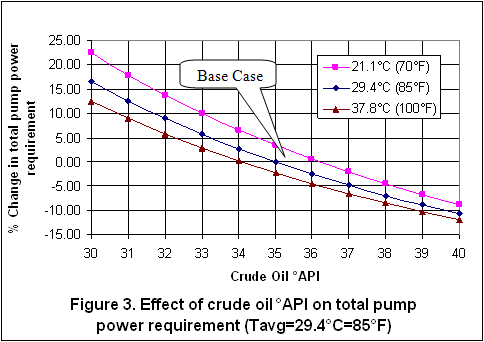
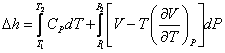

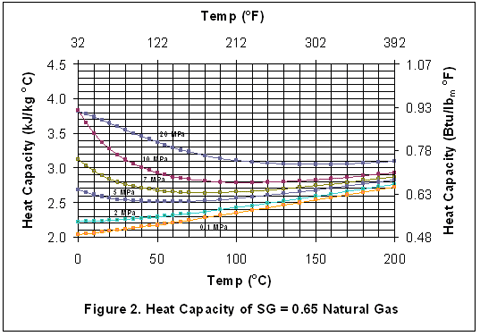
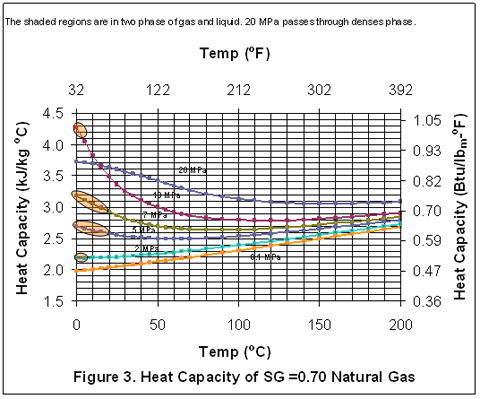
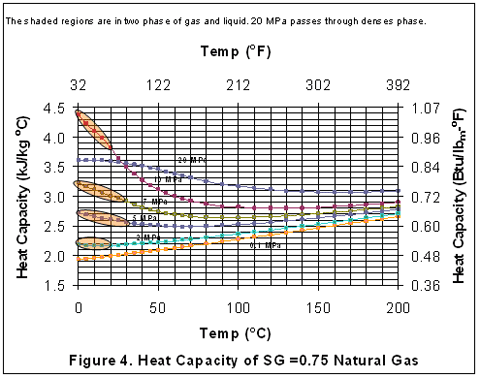

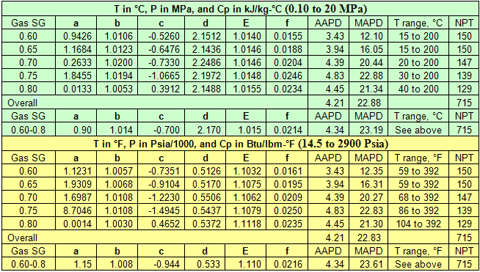
you can tune C6+ weight fractions in several ways, a limit with these methods is that a complete set of vle points is required,
for phase diagrams in addition to Promax and Hysys I would mention also Prode Properties, see http://www.prode.com for a free copy.